![[Shownotes]](../gif/annotate/sshow-11.gif)
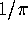 include
include

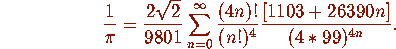
This series adds roughly eight digits per term.
Gosper in 1985 computed 17 million terms of the continued fraction for 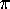 using this.
using this.
Such series exist because various modular invariants are rational (which is more-or-less equivalent to identifying those imaginary quadratic fields of class number 1).
The Chudnovskys' series with d = -163 is

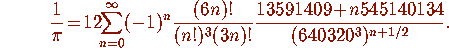
Quadratic versions correspond to class number two imaginary quadratic fields. The largest example has d = -427 and

 where
where

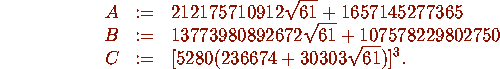
This series adds roughly 25 digits per term. These series are of the form

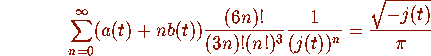 where
where

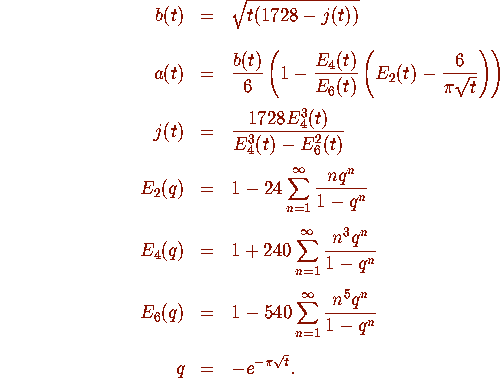
Here t is the appropriate discriminant, j is the ``absolute
invariant'', and 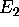 ,
, 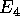 and
and 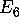 are Eisenstein series.
There is an unlimited number of such series with increasingly more rapid
convergence.
The price is that one must deal with more
complicated algebraic irrationalities.
are Eisenstein series.
There is an unlimited number of such series with increasingly more rapid
convergence.
The price is that one must deal with more
complicated algebraic irrationalities.
A class number p field will
involve 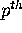 degree algebraic integers as the constants
degree algebraic integers as the constants 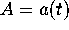 ,
, 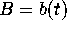 and
and 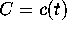 in the series.
in the series.
The largest class number three example with d = -907 gives 37 or 38 digits per term.
The largest class number four example with d = -1555 is

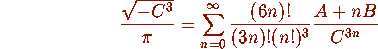
where

 This gives 50 additional digits per
term.
This gives 50 additional digits per
term.
this is discussed in Class number three Ramanujan type series for 1/Pi in J. Comp. and Applied Math. 46 (1993) 281-290.
Deriving the Series
 The absolute invariant,
and so the coefficients A, B, and C satisfy polynomial equations of
known degree and height.
The absolute invariant,
and so the coefficients A, B, and C satisfy polynomial equations of
known degree and height.
 Thus the problem of determining the coefficients
of each series reduces to algebra and can be entirely automated.
Thus the problem of determining the coefficients
of each series reduces to algebra and can be entirely automated.
 From the
expressions for
From the
expressions for 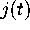 ,
,  ,
, 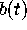 it is easy to compute their
values to several hundred digits.
it is easy to compute their
values to several hundred digits.
 The lattice basis reduction algorithm now provides the minimal polynomials for each
quantity.
The lattice basis reduction algorithm now provides the minimal polynomials for each
quantity.
 In addition, a higher precision calculation actually provides a
proof of the claimed identity.
In addition, a higher precision calculation actually provides a
proof of the claimed identity.
 This last step requires knowing a priori
bounds on the degrees and heights.
This last step requires knowing a priori
bounds on the degrees and heights.