Several authors have investigated the range of validity of the result that

has a finite stopping time for almost all integers n by considering more general classes of periodicity linear functions. One such class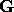

consists of all functions

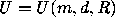which are given by

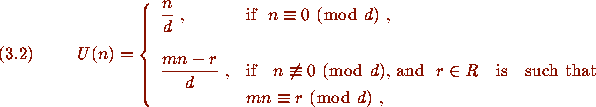where m and d are positive integers with

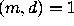and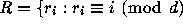

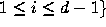is a fixed set of residue class representatives of the nonzero residue classes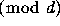

. The 3x+1 function T is in the class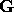

. H. Möller [54] completely characterized the functions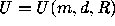

in the set

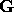which have a finite stopping time for almost all integers n. He showed they are exactly those functions for which

E. Heppner [41] proved the following quantitative version of this result, thereby generalizing Theorem D.

Annotation Form Interface
Your name: E-Mail address: Annotation Subject: Related URL: