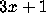Conjecture asserts that all trajectories of positive n are
convergent.
It is certainly true for n > 1 that
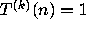cannot occur without some 
occurring.
Call the least
positive k for which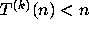 
the
stopping time
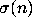of n, and set 
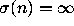if no k occurs
with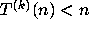 
.
Also call the least positive k for which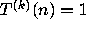 
the total stopping time
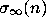of n, and set 
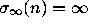if no such k occurs.
We may restate the 
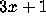Conjecture in terms of the stopping time
as follows.