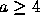Theorem J

(Steiner). The only cycle that is a circuit is the trivial cycle.

Proof J

(sketch). Steiner's method is to show first that any solution of (2.28) with has the property that 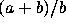is a convergent in the continued fraction expansion of 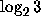, since (2.28) implies that

 

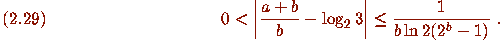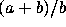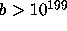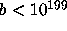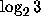He checks that this rational approximation is so good that it violates the effective estimates of A. Baker [[10], p. 45] for linear forms in logarithms of algebraic numbers if . Finally he checks that (2.29) fails to hold for all that by computing the convergents of the continued fractions of up to 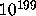.