Frauds

Gregory's series for 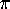, truncated at 500,000 terms gives to forty places

Only the underlined digits are wrong. This is explained by the following Theorem.

Theorem.

For integer N divisible by 4 the following asymptotic expansion holds:

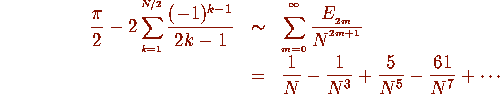where the coefficients are the even Euler numbers 1, -1, 5, -61, 1385, .

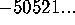Gregory's series requires more terms than there are particles in the universe to compute 100 digits of .

However, with N = 200,000 and correcting using the first thousand even Euler numbers gives over 5,000 digits of .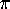

See Pi, Euler numbers and asymptotic expansions by J. Borwein, P. Borwein & K. Dilcher in the MAA Monthly 96 (1989) 681-687.

Excessive Fraud

Sum.

(correct to over 42 billion digits)

The sum arises from an application of Poisson summation or equivalently as a modular transformation of a theta function.

Conjecture.

No one will ever know the

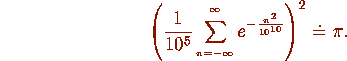
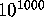th digit of

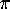(or the

th).