Contents Next: About this document Up: No Title Previous: Appendix 2: Algorithms

Final comments

For Galois cohomology, one might want to know

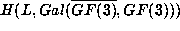, for L the group of order 120 Zhijie mentions on page 25.

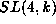In characteristic 0 we have that decomposes into -irreducible as follows, where the square brackets denote high weight modules whose high weight they embrace and exponents indicate multiplicities (bigger than one):

This computation has been done using LiE, cf. [LiE]. The first factor shows that there is a form of degree 8 which is left invariant by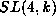

for any field k (not necessarily of characteristic 3). We would like to know of a geometrical explanation of this invariant in terms of cubic surfaces in projective space. Also, it seems reasonable to ask whether, after modding out the invariant 4-dimensional subspace of cubes in the case of characteristic 3, this invariant describes the degree 8 invariant whose existence Chen derived in [Z].

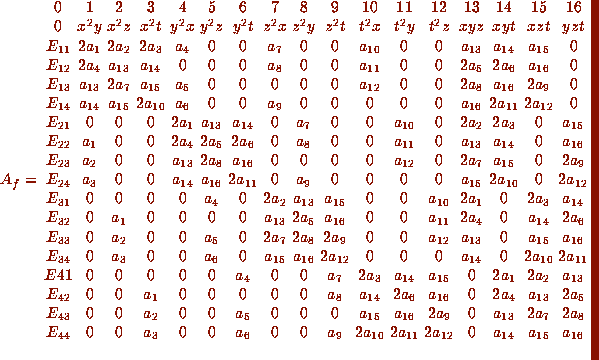
A second method we call the method of determinants. It is used when a form is in but not in 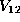. The submatrix would be a nonsingular 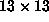matrix so the rank of A would be 13. This means the rows indexed by 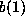are linear combinations of the rows of A included in the submatrix. Suppose the submatrix is 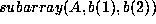. Let 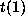be the vector of rows not excluded by and 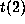the corresponding columns of A not excluded by 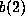. In particular for b above, 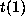would be 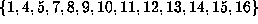and 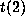would be 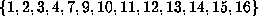. It is straightforward to check that the condition that A has rank 13 is

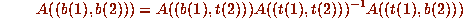where, for

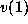and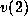

vectors, the notation

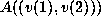is the submatrix of A keeping the rows in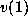

and the columns in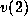

. We denote by D the

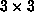matrix above. When we make conclusions using the method of determinants we indicate the entry of D which gives us the information. In our cases, the inverse can always be calculated by cofactors.

Contents Next: About this document Up: No Title Previous: Appendix 2: Algorithms