iii) Transformation Methods

Bailey in January 1986 computed over 29 million digits using Algorithm 1 on a Cray 2 [2]. Kanada [33], using a related quadratic algorithm (due in basis to Gauss and made explicit by Brent [12] and Salamin [25] [27]) and using Algorithm 1 for a check, verified 33,554,000 digits. This employed a HITACHI S--810/20, took roughly eight hours and was completed in September of 1986. In January 1987 Kanada extended his computation to 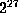decimal places of and the hundred million digit mark had been passed. The calculation took roughly a day and a half on a NEC SX2 machine. Kanada's most recent feat (Jan. 1988) was to compute 201,326,000 digits, which required only six hours on a new Hitachi S-820 supercomputer. Within the next few years many hundreds of millions of digits will no doubt have been similarly computed. Further discussion of the history of the computation of may be found in [5] and [9].