![[Shownotes]](../gif/annotate/sshow-91.gif)
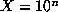 for
for 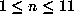 , as well as the elapsed time on a DEC Alpha.
, as well as the elapsed time on a DEC Alpha.
The following table gives the total number of complex fields of discriminant
greater or equal to to -X with  for
for 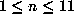 , as well as the
elapsed time on a DEC Alpha.
, as well as the
elapsed time on a DEC Alpha.
It should be remarked that, thanks to the notion of reducedness, our algorithm gives for every cubic field a canonical equation, which we can call reduced, and which in addition has all the nice properties described in Section 4. In particular the integral basis and decomposition of primes is immediate. One consequence is that, when the cubic number field does not have a power basis, the equation that we will find will not be monic. On the other hand, if there exists a power basis, the reduced equation produced by our algorithm is not necessarily monic. We give a few examples.
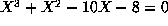 , but does not
have a power basis (an integral basis is
, but does not
have a power basis (an integral basis is 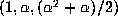 , where
, where  is a root of the above equation). Our algorithm finds the nonmonic reduced
equation
is a root of the above equation). Our algorithm finds the nonmonic reduced
equation 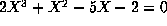 .
.
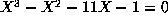 , but does not have a power basis
(an integral basis is
, but does not have a power basis
(an integral basis is 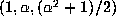 ). Our algorithm finds the nonmonic
reduced equation
). Our algorithm finds the nonmonic
reduced equation 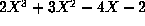 .
.
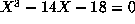 , and this times it has a power basis. On the
other hand our algorithm finds the nonmonic reduced equation
, and this times it has a power basis. On the
other hand our algorithm finds the nonmonic reduced equation
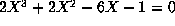 .
.