A note on numerics.

The unique solution in the antifunnel,

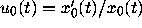, has the value

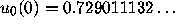. (This is what a standard fourth-order Runge-Kutta approximation will give if you solve backwards from t=-9,u=3 or from

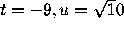; these points are on the top and the bottom of the antifunnel, and thus should give upper and lower bounds for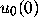

. An error analysis done as in [4, chapter 3], and helped by the program Numerical Methods from MacMath, shows that all written decimal digits of

are correct.) Thus a numerical solution of

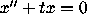, backward in time, starting with

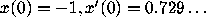, remains bounded for a considerable length of time, as our computer experiments showed---but eventually it will blow up, since the exact solution

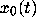is unstable as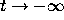