![[Shownotes]](../gif/annotate/shide-51.gif)
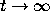 ,
and they are ``straight'' enough to try using the
theory of fences and funnels.
In fact, as a result of Condition II, we see from equation (12) that as
,
and they are ``straight'' enough to try using the
theory of fences and funnels.
In fact, as a result of Condition II, we see from equation (12) that as
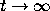 , solutions are going to behave very much like solutions to
the equation
, solutions are going to behave very much like solutions to
the equation 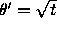 . To be precise, from (12) we get
. To be precise, from (12) we get

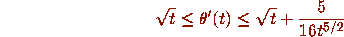 for all t > 0. Therefore, for any C, the curves
for all t > 0. Therefore, for any C, the curves

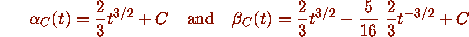 are respectively a lower fence and an upper fence for the differential
equation (12). That is, for every t>0 the slope
are respectively a lower fence and an upper fence for the differential
equation (12). That is, for every t>0 the slope 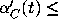 the slope of the direction field for
the slope of the direction field for 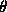 at that point, and
at that point, and
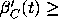 the slope of the direction field. Together these fences
define an antifunnel
the slope of the direction field. Together these fences
define an antifunnel
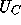 , which is narrowing as
, which is narrowing as 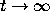 ; see Figure
; see Figure 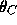 which is trapped in the narrowing antifunnel
which is trapped in the narrowing antifunnel 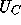 for all
t>0, and that every solution of (12) for t > 0 is of this form for
some C.
for all
t>0, and that every solution of (12) for t > 0 is of this form for
some C.


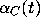 and
and 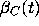 forming the antifunnel
forming the antifunnel 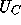 for equation
for equation
 .
.
These facts allow us to determine the spacing of the zeroes of 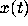 .
Recall that
.
Recall that 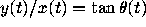 ,
so
,
so  if and only if
if and only if 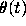 is an odd multiple of
is an odd multiple of 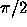 .
Figure
.
Figure ![]() shows the
t-intervals in which the zeros of
shows the
t-intervals in which the zeros of 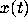 must lie;
that is, the intervals between the curves
must lie;
that is, the intervals between the curves 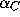 and
and  corresponding to equally spaced values of
corresponding to equally spaced values of  .
.


Figure 6: The t-intervals trapping the zeroes of 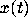 ; here
; here
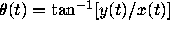 .
.
Let 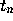 denote the zero of
denote the zero of 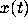 correponding to
correponding to 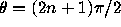 . Since the solution
. Since the solution 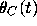 stays inside the
antifunnel
stays inside the
antifunnel 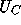 , it is clear that the interval defining
, it is clear that the interval defining 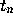 shrinks
as
shrinks
as 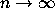 ; in fact, for large n we have
; in fact, for large n we have

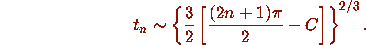 Hence (l'Hôpital) the distance
Hence (l'Hôpital) the distance 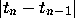 between successive zeros
approaches
0 as
between successive zeros
approaches
0 as 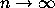 . So our intuition that the oscillations are more and
more compressed, and that the distance between successive zeros goes to
zero as
. So our intuition that the oscillations are more and
more compressed, and that the distance between successive zeros goes to
zero as 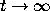 , was correct!
, was correct!